
MATHEMATICA TAYLOR EXPANSION SERIES
In what follows, we illustrate a procedure of this type, based on series expansions for functions of a real variable. Since a power series converges within a symmetrical interval \( \left\vert x - x_0 \right\vert < R, \) it is usually referred to as a local method. Solutions are also determined by the the initial conditions imposed at one single point. This makes Taylor series anĪppropriate technique for solving initial value problems because So only infinitesimal knowledge of theįunction at one single point is needed to find all coefficients of the corresponding power series. Second, when a function is represented (orĪpproximated) by a power series, all its coefficients are determined byĭerivatives evaluated at one point. First of all, historically speaking power series were the first tool toĪpproximate functions, and this topic is part of theĬalculus course. Produce an approximate solution, of which we choose the form of an In such situations, we need to resort to methods that Solution of an initial value problem in a reasonable form. Owing to the complicated structure of some ordinary differentialĮquations, it is not always possible to obtain the corresponding Return to the main page for the course APMA0340 Return to the main page for the course APMA0330 Return to Mathematica tutorial for the second course APMA0340 Return to Mathematica tutorial for the first course APMA0330 Return to computing page for the second course APMA0340 Return to computing page for the first course APMA0330
MATHEMATICA TAYLOR EXPANSION SOFTWARE
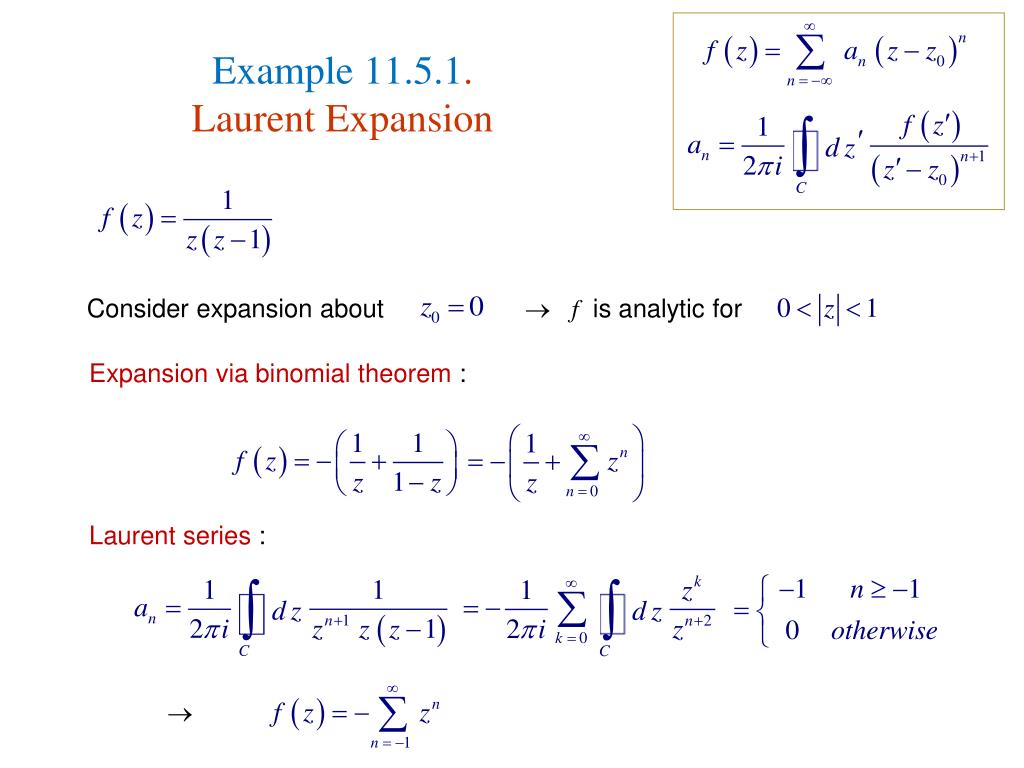
Such jargon is mostly appropriate for users whoĪre involved in the elaboration of software packages-numerical Value parameters) is sometimes referred to as the differential Series of the solution to differential equations (in terms of the initial It should be noted that a technique for (analytically) calculating the power The word derives from the Greek ο λ ο σ (holos), meaning "whole," and μ ο ρ φ η (morphe), meaning "form" or "appearance." The set of functions represented by power series, called the holomorphic functions, is denoted by 𝓗(𝑎, b) and it is a proper subset of C ∞(𝑎, b) of infinitely differentiable functions on the interval (𝑎, b).
The majority of this chapter is devoted to determination of solutions in terms of convergent power series. Also, when the problem is cast in this way, many of the properties of the solution can be investigated without explicitly solving the differential equation. The iteration procedure is conceptually very basic, and sets the tone for a large class of numerical integration techniques. There are several methods to obtain a power series solution, and they all lead to some iteration schemes and solving recurrences. Representing/approximating solutions to nonlinear and linear variable coefficient differential equations as power series.


Series can expand about the point x= ∞.This chapter gives an introduction to recurrences and.On makes Series generate a message in this case. Series detects certain essential singularities.Series can construct standard Taylor series, as well as certain expansions involving negative powers, fractional powers, and logarithms.


 0 kommentar(er)
0 kommentar(er)
